Trig Functions

Section 2.4 Trig Functions

Let's review the unit circle:

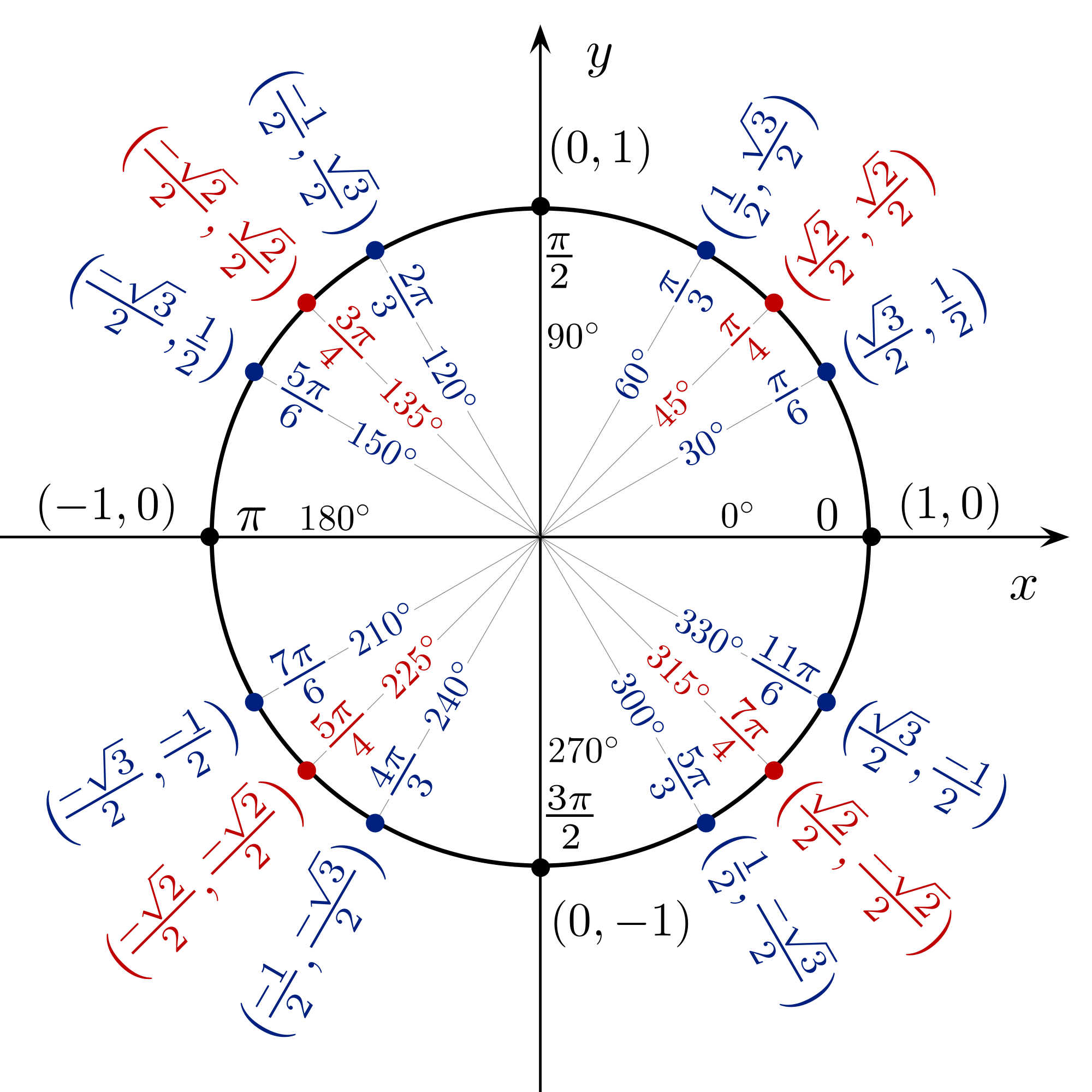
A point on the circle has coordinates $(x, y) = (\cos (θ), \sin (θ)) .$

$(\frac{\sqrt{4}}{2}, \frac{\sqrt{0}}{2}) = 2 π or 0$
$(\frac{\sqrt{3}}{2}, \frac{\sqrt{1}}{2}) = \frac{π}{6}$
$(\frac{\sqrt{2}}{2}, \frac{\sqrt{2}}{2}) = \frac{π}{4}$
$(\frac{\sqrt{1}}{2}, \frac{\sqrt{3}}{2}) = \frac{π}{3}$
$(\frac{\sqrt{0}}{2}, \frac{\sqrt{4}}{2}) = π$

To calculate derivatives, all we know is the limit definition. Let's calculate the derivative of

\cos (x) :

\begin{aligned} \frac{d}{d x} (\cos (x)) & = lim_{h \to 0} \frac{\cos (x + h) - \cos (x)}{h} \\ = lim_{h \to 0} \frac{\cos (x) \cdot \cos (h) - \sin (x) \cdot \sin (h) - \cos (x)}{h} \\ = lim_{h \to 0} - \cos (x) (\frac{1 - \cos (h)}{h}) - \sin (x) (\frac{1 - \sin (h)}{h}) \\ = - \cos (x) (lim_{h \to 0} \frac{1 - \cos (h)}{h}) - \sin (x) (lim_{h \to 0} \frac{1 - \sin (h)}{h}) \\ = - \cos (x) (0) - \sin (x) (1) \\ = - \sin (x) \end{aligned}

Similarly,

\frac{d}{d x} (\sin (x)) = \cos (x)

Let's try finding the derivative of

\tan (x)

using the quotient rule:

\begin{aligned} \frac{d}{d x} (\tan (x)) & = \frac{d}{d x} (\frac{\sin (x)}{\cos (x)}) \\ = \frac{\cos (x) \cdot \frac{d}{d x} (\sin (x)) - \sin (x) \cdot \frac{d}{d x} (\cos (x))}{(\cos (x))^{2}} \\ = \frac{\cos (x) \cdot \cos (x) - \sin (x) (- \sin (x))}{(\cos (x))^{2}} \\ = \frac{\cos^{2} (x) + \sin^{2} (x)}{\cos^{2} (x)} \\ = \frac{1}{\cos^{2} (x)} \\ = \sec^{2} (x) \end{aligned}

Similarly,

\frac{d}{d x} (\cot (x)) = - \csc^{2} (x)

So far:

Table 2.4.2. Trig Function Derivatives

\frac{d}{d x} (\sin (x)) = \cos (x)

\frac{d}{d x} (\cos (x)) = - \sin (x)

\frac{d}{d x} (\tan (x)) = \sec^{2} (x)

\frac{d}{d x} (\cot (x)) = - \csc^{2} (x)

\frac{d}{d x} (\sec (x)) = \sec (x) \cdot \tan (x)

\frac{d}{d x} (\csc (x)) = - \csc (x) \cdot \cot (x)

Derivatives of the "co-" funtions are all negative in sign.

Figure 2.4.4. Slopes

The value of

\cos (x)

describe the slopes of

\sin (x) .

Similarly, we could consider the graph of

\cos (x);

values of

- \sin (x)

describe slopes of

\cos (x) .